QUANTUM FIELDS IN CURVED SPACETIME
My recent research with Tim Hollowood on quantum field theory in curved spacetime has focused on the impact of spacetime geometry on fundamental principles of quantum theory, notably the realisation of causality and unitarity in theories apparently exhibiting superluminal propagation.
This has led to the discovery or improved understanding of many key effects of spacetime geometry in QFT which arise only at quantum loop level, including: (i) the essential role of the Penrose limit in the analysis of quantum propagation, (ii) a novel analytic structure of Green functions arising from the geometry of geodesic congruences, violating several fundamental assumptions of S-matrix theory and dispersion relations, (iii) the existence of below-threshold decays, and (iv) the possibility of real-time renormalization of quantum fields propagating in gravitational backgrounds.
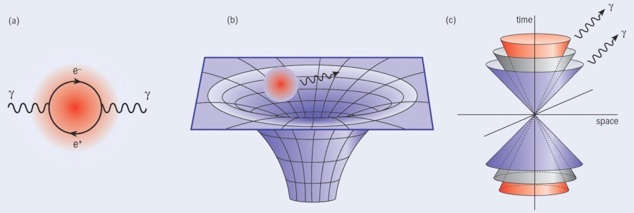
The effect of gravitational tidal forces acting on the virtual cloud of electron-positron pairs surrounding a photon propagating in curved spacetime has been studied in detail [1, 2, 3, 4]. This endows spacetime with a non-trivial refractive index exhibiting remarkable properties unique to gravitational theories, including superluminal low-frequency propagation, in apparent violation of causality, and amplification of the photon field amplitude, in apparent violation of unitarity. The latter effect necessitated the reformulation of the optical theorem in curved spacetime [4], showing that the usual positivity constraint on the imaginary part of amplitudes, normally considered an essential consequence of unitarity, only holds globally while locally manifest positivity is lost. This has potentially wide-reaching implications for quantum theories involving gravity. Our review [5] of this work, focusing on the behaviour of quantum fields near a spacetime singularity, was awarded Third Prize in the 2012 Gravity Research Foundation Essay Competition.
My present work remains focused on how vacuum polarization loop effects cause QFTs to be sensitive to features of spacetime geometry going beyond traditional QFT in curved spacetime. A natural step would be to generalise these results for particle propagation to scattering amplitudes, which will inevitably show new geometry-induced analytic properties violating conventional axioms of S-matrix theory and the optical theorem. Other current and future research topics include:
(i) These novel geometric features depend on the Penrose limit and motivate a study of Cahen-Wallach spaces in the context of AdS x S backgrounds in string theory. These Penrose limits exhibit a coset space structure which can be exploited to gain further insight into QFTs on AdS x S and how to interpret the effects in the dual gauge theory.
(ii) Below-threshold decays are a novel feature of QFT in curved spacetime and together with back-reaction effects may be used to analyse the possibility that quantum instabilities in particle production in de Sitter space may provide a dynamical mechanism for the suppression of the cosmological constant.
(iii) An outstanding question in particle cosmology is the origin of the matter-antimatter symmetry in the universe. Research is underway to investigate the role of quantum loop effects on neutrino physics in curved spacetime and possible mechanisms of gravitational leptogenesis.
(iv) A key area of future research concerns how locality is affected by spacetime geometry, testing the limits of local QFT in situations where fundamental questions concerning quantum mechanics and the non-locality of quantum gravity are expected to arise. This includes QFT/string theory and the nature of black hole and de Sitter horizons, Planck energy scattering, and near-singularity effects.
LORENTZ, CPT and EQUIVALENCE PRINCIPLE VIOLATION
There are clear parallels between the strong equivalence principle violating nteractions in low-energy effective actions induced by quantum loops in curved spacetime and those considered in phenomenological models of Lorentz and CPT violation [6]. Research is continuing on Lorentz, CPT and equivalence principle violating theories from both a fundamental perspective and in the light of their relevance to precision antimatter experiments at CERN, including those designed to measure the gravitational interactions of antimatter directly.
RENORMALISATION GROUP and the c and a-THEOREMS
Techniques developed for quantum field theories in curved spacetime are essential in investigations of general properties of renormalisation group flow even in flat spacetime, and especially the role of the a-theorem, where recently proposed proofs depend in an essential way on positivity and analyticity properties of energy-momentum tensor Green functions implied by the optical theorem [7].
ANOMALIES and QCD PHENOMENOLOGY
Recent work [8] on the phenomenological consequences of the axial U(1) anomaly focused on the potential of proposed e+e- colliders such as Super-B for studying polarized two-photon physics in QCD. In particular, it was demonstrated by explicit calculations of cross-sections and experimental cuts how it would be possible to test the first moment sum rule for the polarized photon structure function first proposed in collaboration with Narison and Veneziano in 1993.
Selected References:
1. The Refractive index of curved spacetime: The Fate of causality in QED,
T.J. Hollowood and G.M. Shore, Nucl. Phys. B795 (2008) 138 [arXiv:0707.2303 [hep-th]].
2. The Causal Structure of QED in Curved Spacetime: Analyticity and the Refractive Index'',
T.J. Hollowood and G.M. Shore, JHEP 0812 (2008) 091 [arXiv:0806.1019 [hep-th]].
3. The Refractive Index of Curved Spacetime II: QED, Penrose Limits and Black Holes,
T.J. Hollowood, G.M. Shore, and R.J. Stanley, JHEP 0908 (2009) 089 [arXiv:0905.0771 [hep-th]].
4. The Effect of Gravitational Tidal Forces on Renormalized Quantum Fields,
T.J. Hollowood and G.M. Shore, JHEP 1202 (2012) 120 [arXiv:1111.3174 [hep-th]].
5. The Unbearable Beingness of Light: Dressing and Undressing Photons in Black Hole Spacetimes,
T.J. Hollowood and G.M. Shore, Int. J. Mod. Phys. D21 (2012) 1241003 [arXiv:1205.3291 [hep-th]].
6. Strong Equivalence, Lorentz and CPT Violation, Antihydrogen Spectroscopy and Gamma Ray Burst Polarimetry,
G.M. Shore, Nucl. Phys. B717 (2005) 86 [hep-th/0409125].
7. The c and a-theorems in two and four dimensions,
G.M. Shore, (in preparation).
8. Polarized Structure Functions and Two-Photon Physics at Super-B,
G.M. Shore, Eur. Phys. J. C73 (2013) 2340 [arXiv:1211.3922 [hep-ph]].